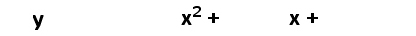A quadratic inequality in two variables is any inequality that can be put into one of the following forms:

• y ≤ ax² + bx + c   (a ≠ 0).
• y < ax² + bx + c   (a ≠ 0).
• y ≠ ax² + bx + c   (a ≠ 0).
• y > ax² + bx + c   (a ≠ 0).
• y ≥ ax² + bx + c   (a ≠ 0).

A quadratic inequality in one variable is any inequality that can be put into one of the following forms:

• 0 ≤ ax² + bx + c   (a ≠ 0).
• 0 < ax² + bx + c   (a ≠ 0).
• 0 ≠ ax² + bx + c   (a ≠ 0).
• 0 > ax² + bx + c   (a ≠ 0).
• 0 ≥ ax² + bx + c   (a ≠ 0).

Recall that inequalities involving "<", "≠" and ">", are called strict inequalities, while inequalities involving "≤" or "≥" are not.

PROBLEM

Graph y > x² - 4x + 3

SOLUTION

Step 1

If the inequality is strict, graph y = x² - 4x + 3 using a dotted line, otherwise graph it using a solid line. Click here to see the steps.

Step 2

Choose a "test" location that is not part of the graph of the equality y = x² - 4x + 3. If the origin is not part of the graph of the equality, it is a "good" location to choose. Substitute the values associated with the test location into the inequality.

y > x² - 4x + 3                Substitute ( 0, 0 ) into the quadratic inequality

(0) > (0)² - 4(0) + 3       Simplify

0 > 3                              (FALSE)

Step 3

If the result is true, shade the region containing the test location. If the result is false, shade the region that does NOT contain the test location. Since ( 0, 0 ) yields a FALSE result, and ( 0, 0 ) is in the exterior of the parabola, the interior of the parabola is shaded.

PROBLEM

Solve and graph x² + 2x - 8 ≤ 0

SOLUTION

Method One: Graph the corresponding quadratic inequality in two variables x² + 2x - 8 ≤ y [or y ≥ x² + 2x - 8]

Method Two: Solve x² + 2x - 8 = 0 , then select appropriate test values for substitution into x² + 2x - 8 ≤ 0

Method Three: Solve x² + 2x - 8 ≤ 0 algebraically.

PROBLEM

Solve and graph x² - 4x + 1 > 0

SOLUTION

Method One: Graph the corresponding quadratic inequality in two variables x² - 4x + 1 > y [or y < x² - 4x + 1]

Method Two: Solve x² - 4x + 1 = 0 , then select appropriate test values for substitution into x² - 4x + 1 > 0

Method Three: Solve x² - 4x + 1 > 0 algebraically.

The applet will graph all of the inequalities described in the definition as well as the corresponding quadratic function y = ax² + bx + c   (a ≠ 0).